Point Of Discontinuity Removable Or Nonremovable - Continuity And Discontinuity Ck 12 Foundation - X = , x = 0 9) removable discontinuity at:

Point Of Discontinuity Removable Or Nonremovable - Continuity And Discontinuity Ck 12 Foundation - X = , x = 0 9) removable discontinuity at:. X = infinite discontinuities at: If a term doesn't cancel, the discontinuity at this x value corresponding to this term for which the denominator is zero is nonremovable, and the graph has a vertical asymptote. F (x) = hon 5+3 cliq covtå non. X = 7) removable discontinuity at: In this case, all limits exist.

Discontinuity meaning in maths, often there are functions f (x) that are not continuous at a point of its domain d. A point of discontinuity is said to beremovable if the limit of a function at that point exists, so that we can replace the existingvalue by the limit value and remove the discontinuity. Is the point of discontinuity. To find the value, plug in into the final simplified equation. X = 7) removable discontinuity at:

Mathwords Removable Discontinuity from www.mathwords.com

A removable discontinuity looks like a single point hole in the graph, so it is removable by redefining f (a) equal to the limit value to fill in the hole. We can simply say that the value of f (a) at the function with x = a (which is the point of discontinuity) may or may not exist but the limit xa f (x) does not exist. Find out information about nonremovable discontinuity. Recall that a function f (x) is continuous at a if For the functions listed below, find the x values for which the function has a removable discontinuity. Lim xa f (x) does not exist. A point at which a function is not continuous or is undefined, and cannot be made continuous by being given a new value at the point. A function is said to be discontinuous at a point when there is a gap in the g.

Derivative is undefined at that point.

The graph of a removable discontinuity leaves you feeling empty, whereas a graph of a nonremovable discontinuity leaves you feeling jumpy. Thus, since lim x→a f(x) does not exist therefore it is not possible to redefine the function in any way so as to make it continuous. If a function has a discontinuity or a sharp point at a particular value of x. Is the point of discontinuity. Discontinuities for which the limit of f(x) exists and is finite are called removable discontinuities for reasons explained below. A removable discontinuity occurs precisely when the left hand and right hand limits exist as equal real numbers but the value of the function at that point is not equal to this limit because it is another real number. X = 10) removable discontinuity at: A removable discontinuity looks like a single point hole in the graph, so it is removable by redefining f (a) equal to the limit value to fill in the hole. X = 8) removable discontinuity at: Wataru · · sep 20 2014 how do you determine removable discontinuity for a function? Discontinuity meaning in maths, often there are functions f (x) that are not continuous at a point of its domain d. This use is abusive because continuity and discontinuity of a function are concepts defined only for points in the function's domain. Removable discontinuities are characterized by the fact that the limit exists.

(often jump or infinite discontinuities.) A point of discontinuity is said to beremovable if the limit of a function at that point exists, so that we can replace the existingvalue by the limit value and remove the discontinuity. If a function has a discontinuity or a sharp point at a particular value of x. But f(a) is not defined or f(a) l. X = 7) removable discontinuity at:

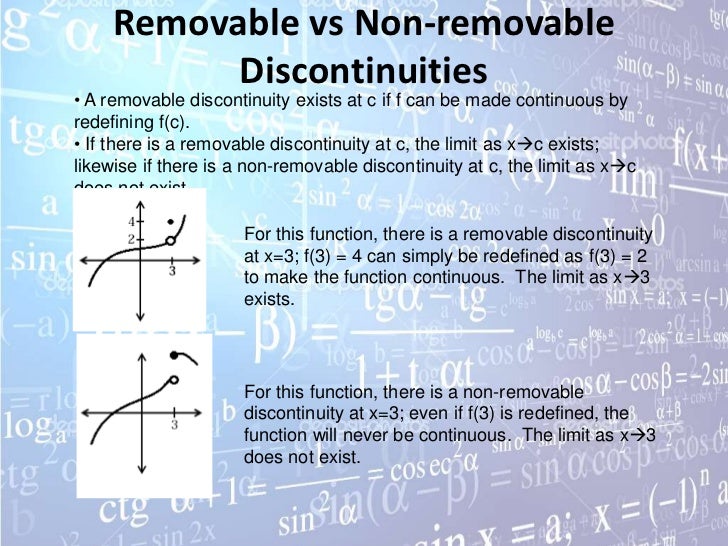
21 Images Removable Discontinuity from image.slidesharecdn.com

Lim xa f (x) does not exist. F (x) = hon 5+3 cliq covtå non. X2 + x— 12 8.f(x) = x2 — 2x — 15 sin x 10. X = 4) removable discontinuity at: A point at which a function is not continuous or is undefined, and cannot be made continuous by being given a new value at the point. A removable discontinuity occurs precisely when the left hand and right hand limits exist as equal real numbers but the value of the function at that point is not equal to this limit because it is another real number. A discontinuity is a point at which a mathematical function is not continuous. Wataru · · sep 20 2014 how do you determine removable discontinuity for a function?

The function is not continuous because there is a jump or because there is an infinite portion which leads to an asymptote.

A removable discontinuity occurs precisely when the left hand and right hand limits exist as equal real numbers but the value of the function at that point is not equal to this limit because it is another real number. The first way that a function can fail to be continuous at a point a is that. The graph of a removable discontinuity leaves you feeling empty, whereas a graph of a nonremovable discontinuity leaves you feeling jumpy. The term removable discontinuity is sometimes an abuse of terminology for cases in which the limits in both directions exist and are equal, while the function is undefined at the point x0. $\begingroup$ @mattsamuel the fact that the op said a removable and nonremovable discontinuity, if read strictly would be a single point that is both since a and discontinuity are singular. Recall that a function f (x) is continuous at a if A removable discontinuity is a point on the graph that is undefined or does not fit the rest of the graph. Informally, the graph has a hole that can be plugged. Thus, since lim x→a f(x) does not exist therefore it is not possible to redefine the function in any way so as to make it continuous. A point of discontinuity is said to beremovable if the limit of a function at that point exists, so that we can replace the existingvalue by the limit value and remove the discontinuity. A function is said to be discontinuous at a point when there is a gap in the g. Discontinuities can be classified as jump, infinite, removable, endpoint, or mixed. But f(a) is not defined or f(a) l.

This calculus video tutorial provides a basic introduction into to continuity. The other types of discontinuities are characterized by the fact that the limit does not exist. X2 + x— 12 8.f(x) = x2 — 2x — 15 sin x 10. Derivative is undefined at that point. The term removable discontinuity is sometimes an abuse of terminology for cases in which the limits in both directions exist and are equal, while the function is undefined at the point x0.

A point at which a function is not continuous or is undefined, and cannot be made continuous by being given a new value at the point. Hole, lim x→c f(x) exists but lim x→c f(x) not equal to f(c). Therefore, we need to know what a removable discontinuity is. X = 7) removable discontinuity at: A removable discontinuity occurs precisely when the left hand and right hand limits exist as equal real numbers but the value of the function at that point is not equal to this limit because it is another real number. But f(a) is not defined or f(a) l. Derivative is undefined at that point. X = 4) removable discontinuity at:

X = infinite discontinuities at:

The other types of discontinuities are characterized by the fact that the limit does not exist. A function is said to be discontinuous at a point when there is a gap in the g. A function having a finite number of jumps in a given interval i is called a piece wise continuous or sectionally continuous function in this interval. X = 4) removable discontinuity at: The term removable discontinuity is sometimes an abuse of terminology for cases in which the limits in both directions exist and are equal, while the function is undefined at the point x0. Discontinuity meaning in maths, often there are functions f (x) that are not continuous at a point of its domain d. For the functions listed below, find the x values for which the function has a removable discontinuity. A point of discontinuity is said to beremovable if the limit of a function at that point exists, so that we can replace the existingvalue by the limit value and remove the discontinuity. Discontinuities for which the limit of f(x) exists and is finite are called removable discontinuities for reasons explained below. X = , x = 0 9) removable discontinuity at: To find the value, plug in into the final simplified equation. It explains the difference between a continuous function and a discontinuous. If a function has a discontinuity or a sharp point at a particular value of x.

Cari Blog Ini

Sosis